
As discussed in §6.1.4, spectra within groups at the same echelle setting sometimes exhibited displacements with respect to each other. These displacements were generally of two types. Either the shift was small and along only the x direction, or they were larger and along a diagonal in +x and +y direction. Their occurrences were unpredictable, much like earthquakes: sometimes there was no movement at all, and at other times the movement was substantial. The frequency and magnitude of the shifts were generally smaller for echelle position 1. Fig. 10 shows the distribution of these movements in x and y.
We never had much difficulty measuring the amount of the shift in y,
since all of the echelle orders would move vertically. Shifts in the
horizontal direction could be measured from the displacements of sharp,
interstellar features. Only in the case of ![]() Eri did we have
difficulty in seeing any lines that could indicate the magnitudes and
directions of shifts in x.
Eri did we have
difficulty in seeing any lines that could indicate the magnitudes and
directions of shifts in x.
While we could compensate for the shifts between successive exposures by
just moving the images as they were added together, there was nothing we
could do about the loss of resolution caused by any movement that might
have occurred during an exposure. There is no easy way to judge this
degradation in the x direction, because one does not know whether the
breadth of any particular absorption feature is intrinsic or caused by
smearing from the instrument. (In principle, one could compare images,
but usually the signal-to-noise ratios in individual cases were not good
enough for this purpose.) We could, however, make fairly accurate
measures of smearing in the y direction, because of the effect on
vertical profiles of all the echelle orders. The slightly
non-sinusoidal shape of the vertical cuts through the orders (see
Fig. 12) meant that some energy could be detected in the
second harmonic of the y component of an image's Fourier transform
(barely visible in Fig. 5 -- the peaks due to the orders are
enhanced if the picture is distorted so that the orders are equidistant
from each other, as discussed in §7.10.1).
By comparing the
power in the second harmonic to that in the first, we could calculate
the amount of smearing, assuming that it occurred about half way through
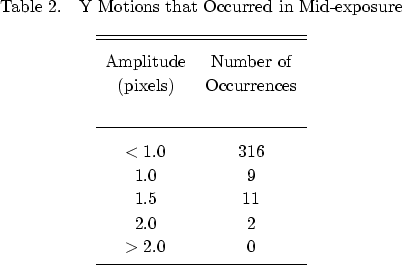
the exposure.![]() Table 2
summarizes our measurements of this effect for a large fraction of the
useful exposures. Statistically, the magnitude and frequency of these
motions are consistent with the motions between exposures, after one
accounts for the disparity between the length of an exposure (34 s) and
the interval from one exposure to the next (99 s). On the basis of the
general properties of the motions shown in Fig. 10, it is
probably safe to assume that if an image's y motion is small, its x
movement is probably less than about 4 pixels (or 2 of the CCD's
30 µm pixels), and, in a good fraction of the cases, 0 pixels.
Table 2
summarizes our measurements of this effect for a large fraction of the
useful exposures. Statistically, the magnitude and frequency of these
motions are consistent with the motions between exposures, after one
accounts for the disparity between the length of an exposure (34 s) and
the interval from one exposure to the next (99 s). On the basis of the
general properties of the motions shown in Fig. 10, it is
probably safe to assume that if an image's y motion is small, its x
movement is probably less than about 4 pixels (or 2 of the CCD's
30 µm pixels), and, in a good fraction of the cases, 0 pixels.